| Example: Find the eigenvalues and the eigenvectors of the matrix A = |
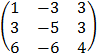 |
| From the eigenvalues equation we get the characteristic polynomial: |
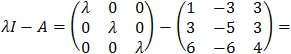 |
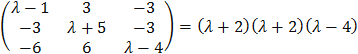 |
The eigenvalues are the roots of the characteristic polynomial, and are:
− 2, − 2, 4 this values are the diagonal values and has the same determinant
value as matrix A. In order to find the eigenvectors, substitute first solution
λ =− 2 into the characteristic matrix, the result is:
| Those equations reduce to one independent equation: |
x − y + z = 0 |
| Choose arbitrary y = 0 to receive the first eigenvector |
x = 1, y = 0, z = − 1 |
| Choose arbitrary z = 0 to get the second vector: |
x = 1, y = 1, z = 0 |
Perform the same process with the third solution λ = 4 to get:
| The result is two independent equations: |
x + y − z = 0 and 2y − z = 0 |
| Choose arbitrary y = 1 to receive the vector |
x = 1, y = 1, z = 2 |
| The three eigenvectors are: |
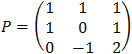 |
We can see that the result is matching to the definition of the eigenvectors and eigenvalues:
|